Question

Svetlana won $1,000,000 in a contest, to be paid in twenty $50,000 payments at yearly intervals,the rst payment paid at the time of the contest. (Of course, the present value of her winningis less than $1,000,000.) Svetlana decided to keep X each year to spend and deposit theremaining $50,000-X into an account earning an annual eective interest rate of 5%. Shechose the value X to be as large as possible so that, at the moment of the 20th deposit, theaccount would have grown to such a size that it would provide Svetlana and her heirs at leastX per year in interest forever. Find X.

Answer 1

The amount X she can retire out of every payment is $31,155.52.

Explanation:

We start calculating the amount needed to provide an perpetuity of X after the 20th deposit. The formula for the the present value of a perpetuity is

`PV=(X)/(i)=(X)/(0.05)=20X`

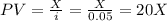
This is the amount of capital she has to have in her account to provide X yearly forever.

We have 20 payments, which are compunded at an effective rate of 5%. The amount deposit out every payment is (50,000-X).

We can write a timeline to see all the deposits

• Year 0: First deposit (50,000-X)
• Year 1: Second deposit (50,000-X)
• ...
• Year 19: Last deposit (50,000-X). By this time, the capital in the account should be 20X.

We can express then the capitalization of the deposit (C) as

`C=\sum\limits^(19)_(k=0) {(50000-X)(1+0.05)^k} \\\\C=(50000-X)\sum\limits^(19)_(k=0) 1.05^k=(50000-X)*33.07=1,653,297-33.07X\\`

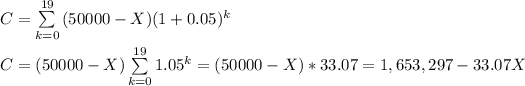
This capital C has to be equal to 20X:

`C=1,653,297-33.07X=20X\\\\1,653,297=53.07X\\\\X==1,653,297/53.07=31,155.52`

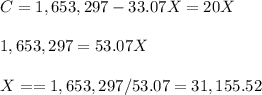
Then, the amount X she can retire out of every payment is $31,155.52.