Question

The average expenditure on Valentine's Day was expected to be $100.89 (USA Today, February 13, 2006). Do male and female consumers differ in the amounts they spend? The average expenditure in a sample survey of 40 male consumers was $135.67, and the average expenditure in a sample survey of 30 female consumers was $68.64. Based on past surveys, the standard deviation for male consumers is assumed to be $35, and the standard deviation for female consumers is assumed to be $20. The z value is 2.576 .

Answer 1

Yes. The male and female consumers differ in the amounts they spend.

Explanation:

We can express the null and alternative hypothesis as:

`H_0: \mu_m=\mu_w\\\\H_1:  \mu_m\\eq\mu_w`

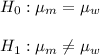
It is assumed a significance level of 0.05.

The standard deviation of the difference of means is calculated as:

`s=\sqrt{(s_m^2)/(n_m) +(s_w^2)/(n_w) } =\sqrt{(35^2)/(40) +(20^2)/(30) } =√(30.625+13.333) =√(43.958) =6.63`

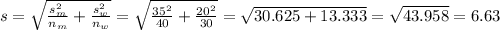
The test statistic is

`t=((M_m-M_w)-0)/(s)=(135.67-68.64)/(6.63)=10.11`

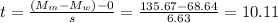
The degrees of freedom are:

`df=n_1+n_2-2=40+30-2=68`

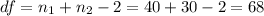
The P-value for t=10.11 is P=0, so it is smaller than the significance level. The null hypothesis is rejected.

We can conclude that male and female consumers differ in the amounts they spend.