Question

All 231 students in math club went on a field trip some rode in vans that held 7 people and some rode on buses that held 25 people if it was 15 total vehicles how many of each type was it

Answer 1

8 vans

7 buses

Explanation:

Let there be "b" buses

and "v" vans

Since 7 people is the capacity of vans, the total capacity is

7v

Also, since 25 people is the capacity of 1 bus, the total capacity is

25b

In total 231 people, so we can write our first equation as:

7v + 25b = 231

Now, we know there are 15 vehicles (bus + vans) in total, so we can write our 2nd equation as:

v + b = 15

Now, we solve for v and b. Let's solve the 2nd equation for v and substitute that into 1st and solve for b first:

v + b = 15

v = 15 - b

Now,

`7v + 25b = 231\\7(15-b) + 25b = 231\\105-7b+25b=231\\18b=126\\b=7`

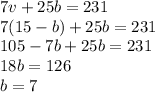
Hence, there are 7 buses

Since 15 vehicles in total, the number of vans is:

15 - 7 = 8 vans

So,

8 vans

7 buses