Answer:
20.76 units
Explanation:
The distance between the points (-4,2) and (2,2) is
 units.
units.
Now, distance between the points (2,2) and (2,-1) is
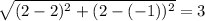 units.
units.
Again the distance between the points (2,-1) and (-2,-3) is
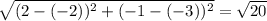 units.
units.
Now, the distance between the points (-2,-3) and (-5,-2) is
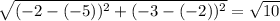 units.
units.
Again the distance between the points (-5,-2) and (-4,2) is
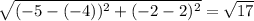
Therefore, the perimeter of the polygon is (6 + 3 + √20 + √10 + √17) = 20.76 units {Rounded to the nearest tenth of the unit} (Answer)