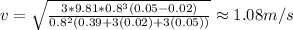Answer:
1.10 m/s
Step-by-step explanation:
Linear speed is given by
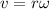
Kinetic energy is given by
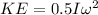
Potential energy
PE= mgh
From the law of conservation of energy, KE=PE hence
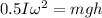 where m is mass, I is moment of inertia,
where m is mass, I is moment of inertia,
 is angular velocity, g is acceleration due to gravity and h is height
is angular velocity, g is acceleration due to gravity and h is height
Substituting m2-m1 for m and 0.5l for h,
 for
for
 we obtain
we obtain
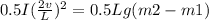
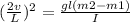 and making v the subject
and making v the subject
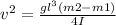
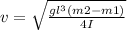
For the rod, moment of inertia
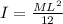 and for sphere
and for sphere
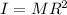 hence substituting 0.5L for R then
hence substituting 0.5L for R then
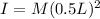
For the sphere on the left hand side, moment of inertia I
 while for the sphere on right hand side,
while for the sphere on right hand side,
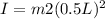
The total moment of inertia is therefore given by adding

Substituting
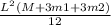 for I in the equation
for I in the equation
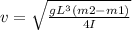
Then we obtain
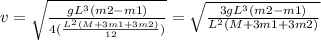
This is the expression of linear speed. Substituting values given we get