Question

Complete each congruence statement by naming the corresponding angle or side (odd questions only)

Answer 1

Given:

11.
`\Delta DCB\cong \Delta CDM`

13.
`\Delta CBA\cong \Delta STA`

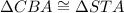
To find:

The missing values to complete the congruence statements:

11.
`\overline{BD}\cong \_\_\_\_\_`

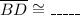
13.
`\angle BAC\cong \_\_\_\_\_`

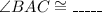
Solution:

11.

We have,

`\Delta DCB\cong \Delta CDM`

Here, vertices D, C, B are corresponding to C, D, M. So,

`\overline{BD}\cong \overline{MC}`

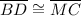
Therefore, the complete statement is
`\overline{BD}\cong \overline{MC}`.

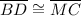13.

We have,

`\Delta CBA\cong \Delta STA`

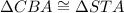
Here, vertices C, B, A are corresponding to S, T, A. So,

`\angle BAC\cong \angle TAS`

Therefore, the complete statement is
`\angle BAC\cong \angle TAS`.