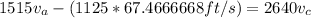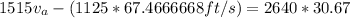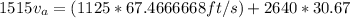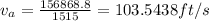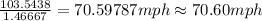Question

you have been called to testify as a as an expert witness in a trial involving a head-on collision Car A weighs 1515 pounds and was traveling Eastward Car B weighs 1125 lb and was traveling Westward at 46 miles per hour. The car locked bumpers and slide eastward with their wheels locked for 19.5 ft before stopping. You have measured the coefficient of kinetic friction between the tires and the pavement to be .750. How fast in miles per hour was car A traveling just before the Collision?​

Answer 1

70.6 mph

Step-by-step explanation:

Car A mass= 1515 lb

Car B mass=1125 lb

Speed of car B is 46 miles/h

Distance before locking, d=19.5 ft

Coefficient of kinetic friction is 0.75

Initial momentum of car B=mv where m is mass and v is velocity in ft/s

46 mph*1.46667=67.4666668 ft/s

`Momentum_B=1125*67.4666668 ft/s`

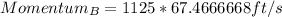
Initial momentum of car A is given by

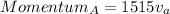`Momentum_A=1515v_a` where
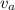`v_a` is velocity of A

Taking East as positive and west as negative then the sum of initial momentum is

`1515v_a-(1125*67.4666668 ft/s)`

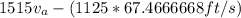
The common velocity is represented as
`v_c` hence after collision, the final momentum is

`Momentum_final=(m_a+m_b)v_c=(1515+1125)v_c=2640v_c`

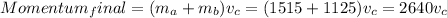
From the law of conservation of linear momentum, sum of initial and final momentum equals each other hence

`1515v_a-(1125*67.4666668 ft/s)= 2640v_c`

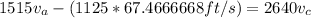
The acceleration of two cars
`a=-\mug=-0.75*32.17=-24.1275 ft/s^(2)`

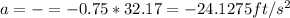
From kinematic equation

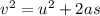`v^(2)=u^(2)+2as` hence

`v^(2)-u^(2)=2as`

`0^(2)-(v_c)^(2)=2*-24.1275*19.5`

`v_c=√(2*24.1275*19.5)=30.67 ft/s`

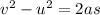
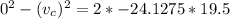
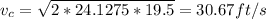
Substituting the value of
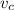`v_c` in equation
`1515v_a-(1125*67.4666668 ft/s)= 2640v_c`

`1515v_a-(1125*67.4666668 ft/s)= 2640*30.67`

`1515v_a=(1125*67.4666668 ft/s)+2640*30.67`

`v_a=\frac {156868.8}{1515}=103.5438 ft/s`

`\frac {103.5438}{1.46667}=70.59787 mph\approx 70.60 mph`