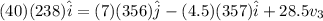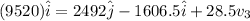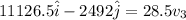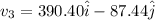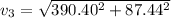Question

A 40.0-$kg$ body is moving in the direction of the positive x axis with a speed of 238 $m/s$ when, owing to an internal explosion, it breaks into three pieces. One part, whose mass is 7.0 $kg$, moves away from the point of explosion with a speed of 356 $m/s$ along the positive y axis. A second fragment, whose mass is 4.5 $kg$, moves away from the point of explosion with a speed of 357 $m/s$ along the negative x axis. What is the speed of the third fragment

Answer 1

`v_3 = 400\ m/s`

Step-by-step explanation:

given,

mass of the body = 40 Kg

speed in x-axis = 238 m/s

mass break into three part

m₁ = 7 kg

v₁ = 356 m/s (along the positive y axis)

m₂ = 4.5 kg

v₂ = 357 m/s(along the negative x axis)

m₃ = 40 - (7 + 4.5) = 28.5 Kg

v₃ = ?

using conservation of momentum

MV = m₁v₁ + m₂v₂ + m₃v₃

`(40)(238) \hat{i} = (7)(356) \hat{j} - (4.5)(357) \hat {i} + 28.5 v_3`

`(9520) \hat{i} = 2492 \hat{j} - 1606.5\hat {i} + 28.5 v_3`

`11126.5 \hat{i} - 2492 \hat{j} = 28.5 v_3`

`v_3 =390.40 \hat{i} - 87.44 \hat{j}`

`v_3 = √(390.40^2 + 87.44^2)`

`v_3 = 400\ m/s`