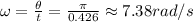Answer:
7.38 rad/s
Step-by-step explanation:
Assume no air resistance, we can first calculate the time it takes for the toast to be dropped 0.89m to the floor. Since we have
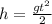
where
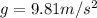 and h = 0.89 m
and h = 0.89 m
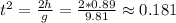
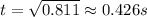
This is also the time for the toast to flip one time at a constant angular speed. The angle it covered would be at
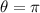 radian.
radian.
So the smallest angular speed it needs to hit and topple butter-side down is