Answer: The plan A would be better deal for more than 300 calling minutes.
Explanation:
Since we have given that
Plan A has a monthly fee of $15 with a charge of $0.08 per minute for all calls
Let the number of minutes be 'x'.
So, Equation would be
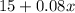
Plan B has a monthly fee of $3 with a charge of $0.12 per minute for all calls.
So, Equation would be
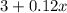
We need to find the number of calling minutes in a month to make plan A the better deal.
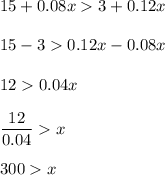
Hence, the plan A would be better deal for more than 300 calling minutes.