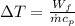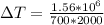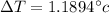Our values are defined by,


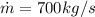
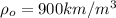
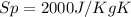
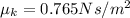
With this data we can easily calculate first the mean velocity of the flow,
The velocity is given by,
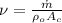
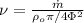
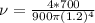
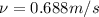
With the mean velocity is now possible determine if the flow is Laminar or turbulent, for that we need Number Reynolds.
Remember that a Laminar Flow is given when Reynolds number is minor to 2300, then
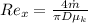
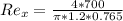
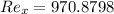
 , then the flow is laminar.
, then the flow is laminar.
For this section we will consider the Pressure drop to calculate the flow Work and the Total Energy conservation of this flow.
To calculate the Pressure drop we need the Friction factor, which is given by,
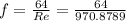
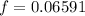
Then the Pressure drop is given by,

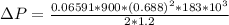
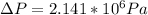
We can now calculate the Flow Work given by,
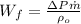
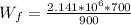
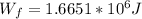
From energy conservation we have
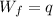

Re-arrange for