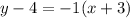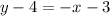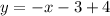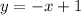Question

Find the midpoint of PQ¯¯¯¯¯¯¯¯ with endpoints P(−7, 0) and Q(1, 8). Then write an equation of the line that passes through the midpoint and is perpendicular to PQ¯¯¯¯¯¯¯¯ . This line is called the perpendicular bisector. The midpoint is ( , ). The equation of the perpendicular bisector is y = .

Answer 1

95

Explanation:

big ideas math answer (trust me)

Answer 2

Answer with Step-by-step explanation:

We are given that

P(-7,0) and Q(1,8)

We have to find the midpoint of PQ and write an equation of the line that passes through the midpoint.

Mid-point formula:
`x=(x_1+x_2)/(2), y=(y_1+y_2)/(2)`

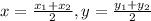
By using this formula ,

The mid point of PQ

`x=(-7+1)/(2)=-3, y=(0+8)/(2)=4`

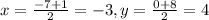
Hence, the midpoint of PQ is (-3,4).

Slope of PQ=
`m=(y_2-y_1)/(x_2-x_1)=(8-0)/(1+7)=1`

Slope of perpendicular line=
`(-1)/(slope\;of PQ)=-1`

The equation of line which is passing through the point (-3,4) with slope -1 is given by

`y-y_1=m(x-x_1)`

The equation of line which is passing through the point (-3,4) with slope -1 is given by

`y-4=-1(x+3)`

`y-4=-x-3`

`y=-x-3+4`

`y=-x+1`