Question

Problem 4.079 SI A rigid tank whose volume is 3 m3, initially containing air at 1 bar, 295 K, is connected by a valve to a large vessel holding air at 6 bar, 295 K. The valve is opened only as long as required to fill the tank with air to a pressure of 6 bar and a temperature of 320 K. Assuming the ideal gas model for the air, determine the heat transfer between the tank contents and the surroundings, in kJ.

Answer 1

`Q_(cv) = -1007.86kJ`

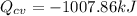
Step-by-step explanation:

Our values are,

State 1

`V=3m^3\\P_1=1bar\\T_1 = 295K`

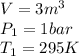
We know moreover for the tables A-15 that

`u_1 = 210.49kJ/kg\\h_i = 295.17kJkg`

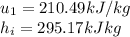
State 2

`P_2 =6bar\\T_2 = 296K\\T_f = 320K`

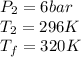
For tables we know at T=320K

`u_2 = 228.42kJ/kg`

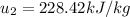
We need to use the ideal gas equation to estimate the mass, so

`m_1 = (p_1V)/(RT_1)`

`m_1 = (1bar*100kPa/1bar(3m^3))/(0.287kJ/kg.K(295k))`

`m_1 = 3.54kg`

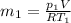
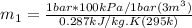
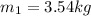
Using now for the final mass:

`m_2 = (p_2V)/(RT_2)`

`m_2 = (1bar*100kPa/6bar(3m^3))/(0.287kJ/kg.K(320k))`

`m_2 = 19.59kg`

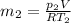
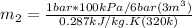
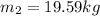
We only need to apply a energy balance equation:

`Q_(cv)+m_ih_i = m_2u_2-m_1u_1`

`Q_(cv)=m_2u_2-m1_u_1-(m_2-m_1)h_i`

`Q_(cv) = (19.59)(228.42)-(3.54)(210.49)-(19.59-3.54)(295.17)`

`Q_(cv) = -1007.86kJ`

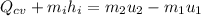
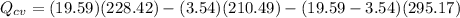
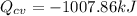
The negative value indidicates heat ransfer from the system