Question

Consider a single crystal oriented such that the slip direction and normal to the slip plane are at angles 42.7° and 48.3°, respectively, with the tensile axis. If the critical resolved shear stress is 28.5 MPa, what applied stress (in MPa) will be necessary to cause the single crystal to yield?

Answer 1

Applied Stress > 58.29 MPa

Step-by-step explanation:

• Resolved shear stress should be greater than critically resolved shear stress in order to cause the single crystal to yield

Given angles are

∅ = 42.7 degree

Ф = 48.3 degree

Critically resolved shear stress = 28.5 MPa

If we consider

Critically resolved shear stress = resolved shear stress

Applied stress can be found by

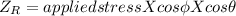`Z_(R) = applied stress X cos\phi X cos\theta` (1)

Applied Stress =
`(Z_(R) )/(Cos\phi XCos\theta)`

Applied Stress =
`(28.5)/(Cos(48.3)XCos(42.7))`

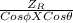
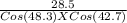
Applied Stress = 58.29 MPa

We got reference

• By putting applied stress values of greater than 58.29 MPa in equation 1 we get

Resolved Shear Stress = 60 x Cos(48.3) x Cos(42.7)

Resolved Shear Stress = 29.33 MPa

Therefore, by the above calculation we conclude that applied stress should be greater than 58.29 MPa, In order to make resolved shear stress to be greater than critically resolved shear stress that is essential for single crystal to yield.