Answer: (-6-
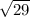 , -6+
, -6+
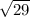 ) ==> D
) ==> D
Explanation:
x^2 + 12x +7 < 0
x^2+12x+36-29<0
(x+6)^2-29<0
(x+6)^2<29
x+6<
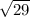
x<-6+
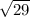
x+6>-
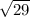
x>-6-
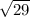
(-6-
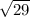 , -6+
, -6+
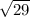 ) ==> D
) ==> D
-6-
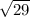 and -6+
and -6+
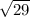 aren't included in the solution since if these values are plugged in to x^2 + 12x +7, the expression will equal 0. That's not supposed to happen. The expression is supposed to be LESS than 0.
aren't included in the solution since if these values are plugged in to x^2 + 12x +7, the expression will equal 0. That's not supposed to happen. The expression is supposed to be LESS than 0.