Question

A firm has a revenue function that can be represented by r (x)=700x-0.35x^2, where r (x) is the total revenue (in dollars) and x is the number of units sold. How many units need to be sold to produce the maximum revenue? How many in dollars is the maximum revenue when the maximum of units are sold?

Answer 1

How many units need to be sold to produce the maximum revenue? 1000 units

How many in dollars is the maximum revenue when the maximum of units are sold? $350,000

Explanation:

We get max value of a function if we differentiate it and set it equal to 0.

We need to differentiate r(x) and set it equal to 0 and solve for x.

That would be number of units sold to get max revenue.

Then we take that "x" value and substitute into r(x) to get the max revenue amount.

Before differentiating, we see the rules shown below:

`f(x)=ax^n\\f'(x)=n*ax^(n-1)`

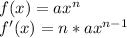
Where

f'(x) is the differentiated function

Now, let's do the process:

`r (x)=700x-0.35x^2\\r(x)=700-2*0.35x\\r(x)=700-0.7x\\0=700-0.7x\\0.7x=700\\x=1000`

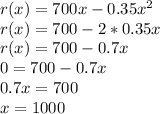
So, 1000 units need to be sold for max revenue

Now, substituting, we get:

`r (x)=700x-0.35x^2\\r(1000)=700(1000)-0.35(1000)^2\\r(1000)=350,000`

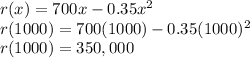
The max revenue amount is $350,000