Question

Find the equation of a line that runs through

(-6,2) and is parallel to a line with a slope of -1/4

Answer 1

The equation of a line that runs through (-6,2) and is parallel to a line with a slope of (-1/4) is x + 4y - 2 = 0

Explanation:

Slope of the parallel equation is m 1 = (-1/4)

If the two liner are parallel the, slope of both lines are equal.

⇒The slope of the equation of line = m2 = m1 = -(1/4)

The point (x0, y0) = (-6,2)

Now, by THE POINT SLOPE FORMULA: The equation of a line is given as

( y - y0) = m (x -x0)

Now, here the equation of line is given as:

`y - 2 = ((-1)/(4)) (x - (-6))   \implies  4(y-2) = -1(x + 6)`

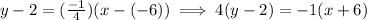
or, 4y - 8 + x + 6 = 0

or, x + 4y - 2 = 0

Hence, the required line equation is x + 4y - 2 = 0