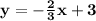Question

Write the equation of the line in slope intercept form that passes through the point (-3, 5) and is parallel to y= - 2/3x

Answer 1

`\displaystyle y = -(2)/(3)x + 3`

Explanation:

5 = –⅔[–3] + b

2

`\displaystyle 3 = b \\ \\ y = -(2)/(3)x + 3`

Parallel equations have SIMILAR RATE OF CHANGES [SLOPES], so –⅔ remains as is.

I am joyous to assist you at any time.

Answer 2

The equation of the line in slope intercept form that passes through the point (-3, 5) and is parallel to y= - 2/3x is
`\mathbf{y=-(2)/(3)x+3 }`

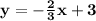
Explanation:

We need to Write the equation of the line in slope intercept form that passes through the point (-3, 5) and is parallel to y= - 2/3x

The equation in slope-intercept form is:
`y=mx+b` where m is slope and b is y-intercept.

Finding Slope:

The both equations given are parallel. So, they have same slope.

Slope of given equation y= - 2/3x is m = -2/3

This equation is in slope-intercept form, comparing with general equation
`y=mx+b` where m is slope , we get the value of m= -2/3

So, slope of required line is: m = -2/3

Finding y-intercept

Using slope m = -2/3 and point (-3,5) we can find y-intercept

`y=mx+b\\5=-(2)/(3)(-3)+b\\5=2+b\\ b=5-2\\b=3`

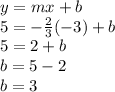
So, we get b = 3

Now, the equation of required line:

having slope m = -2/3 and y-intercept b =3

`y=mx+b\\y=-(2)/(3)x+3`

The equation of the line in slope intercept form that passes through the point (-3, 5) and is parallel to y= - 2/3x is
`\mathbf{y=-(2)/(3)x+3 }`