Question

A strip of copper 150 μm thickand 4.5 mm wide is placed in a uniform magnetic field B (vector) ofmagnitude 0.65 T, with B (vector) perpendicular to the strip. Acurrent i=23 A is then sent through the strip such that aHall potential difference V appears across the widthof the strip. Calculate V. (The number of charge carriersper unit volume for copper is 8.47 x 1028electrons/m3.)

I used V = Bi / nle so V = (.65T*23A)/8.47e28*1.6e-19*150e-5m) = 7.35e-6V which is what the2nd response in the other thread used. And, according to thistextbook, it says "l" is the thickness of the strip however in theother thread, in the first response, they used V = E d where E =1.103e-9 and d = 4.5e-3 therefore the answer is 4.96 e-12

Which is correct?

Answer 1

`V_H=7.3544* 10^(-6) \,V`

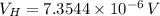
Step-by-step explanation:

Given that:

thickness of the metal strip,
`d=150* 10^(-6) m`

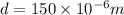
width of the metal strip,
`w=4.5* 10^(-3)m`

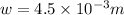
magnitude of the perpendicular magnetic field,
`B=0.65 \,T`

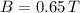
current through the strip,
`I=23\,A`

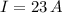
charge density,
`n=8.47* 10^(28) \,electrons\,per\,m^3`

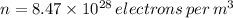
Hall voltage is a transverse voltage given by:

`V_H=(I.B)/(n.e.d)`

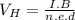
putting the respective values

`V_H=(23* 0.65)/((8.47* 10^(28))* 1.6* 10^(-19)* (150* 10^(-6) ))`

`V_H=7.3544* 10^(-6) \,V`

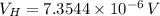
Note:

V = E d is used when a charge is moved in a uniform electric field between the two oppositely charged plates, it can't be used here because it is the case of Hall effect where the small voltage develops transverse to the direction of current flow.