Answer:
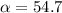 º
º
Step-by-step explanation:
From the exercise we have our initial information
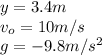
When the balloon gets to the ceiling its velocity at that moment is 0 m/s. Being said that we can calculate velocity at the vertical direction
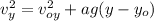
Since
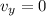 and
and
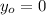

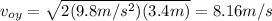
Knowing that
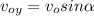
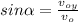
 º
º