Question

What is the point-slope form and standard form of (3,1) and (4, 2)?

Answer 1

The point-slope form and standard form of (3,1) and (4, 2) are y – 1 = x – 3 and x – y = 2 respectively

Solution:

Given, two points are (3, 1) and (4, 2)

We have to find that a line that passes through the given two points.

First let us find the slope of the line that passes through given two points.

Slope of line "m" is given as:

`\mathrm{m}=(y_(2)-y_(1))/(x_(2)-x_(1))`

`\text { where, }\left(x_(1), y_(1)\right) \text { and }\left(x_(2), y_(2)\right) \text { are two points on line. }`

`\text { Here } x_(1)=3 \text { and } y_(1)=1 \text { and } x_(2)=4 \text { and } y_(2)=2`

`\mathrm{m}=(2-1)/(4-3)=(1)/(1)=1`

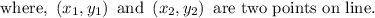
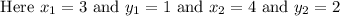
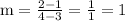
The point slope form is given as:

`y-y_(1)=m\left(x-x_(1)\right)`

`\text { where } m \text { is slope and }(x_1, y_1) \text { is point on the line. }`

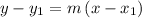
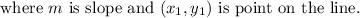
y – 1 = 1(x – 3)

y - 1 = x - 3

Line equation in point slope form is y – 1 = x – 3 -- eqn 1

Now, line equation in standard form i.e. ax + by = c is found out by eqn 1

y – 1 = x – 3

x – y = 3 – 1

x – y = 2

Hence, the line equation in point slope form and standard forms are y – 1 = x – 3 and x – y = 2 respectively