Question

What is the equation of the line that passes through the points (-2, -3) and (4, -5)

Answer 1

x + 3y + 11 = 0 is the required equation.

Explanation:

Here the given points are A( -2,-3) and B(4,-5)

Now, slope of the equation =
`(y_2 - y_1)/(x_2 - x_1)  = (-5 -(-3)))/(4-(-2))   = (-2)/(6)   =-(1)/(3)`

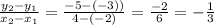
Hence, the slope of the equation is m = (-1/3)

Now, by POINT SLOPE FORMULA:

The equation of a line with slope m and point (x0, y0) is given as

(y - y0) = m (x -x0)

So, here the equation of line with (4, -5) is

`y - (-5) = -(1)/(3)  (x - 4)  \implies  3( y +5) = -x + 4`

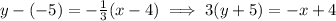
or, 3y + 15 + x-4 = 0

or, x + 3y + 11 = 0

⇒ x + 3y + 11 = 0 is the required equation.