Question

Allie said the degree of the polynomial function


`f(x) = {x}^(5) + 2 {x}^(4) + 3 {x}^(3) - 2 {x}^(6) - 9 {x}^(2) + 6x + 4`
is 5. Explain and correct Allie's error​

Answer 1

Answer:6

Step-by-step explanation: Allie did not write the polynomial function in standard form; instead, she said the exponent of the first term was the degree of the polynomial. The degree is 6

Answer 2

6

Explanation:

Given the polynomial

`f(x)=x^5+2x^4+3x^3-2x^6-9x^2+6x+4.`

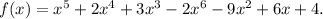
Rewrite it in the standard form (a polynomial is in standard form when its term of highest degree is first, its term of 2nd highest degree is 2nd and so on)

The highest degree is 6, so the first is term
`-2x^6,` then the degrees are 5, 4, 3, 2, 1 and 0, so the polynomial in the standard form is

`f(x)=-2x^6+x^5+2x^4+3x^3-9x^2+6x+4.`

The degree of the polynomial is the highest of the degrees of its terms, so the degree of this polynomial is 6. Allie is incorrect, he didn't notice
`-2x^6` term.