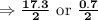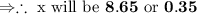2 Answers

Silversonic · Answer 1 · 2020-03-27T08:30:08+0000

The value of x will be 8.65 or 0.35

Solution:

Given,
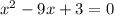
x^2-9x+3=0

Here, to solve the given equation, we have to use the quadratic formula,

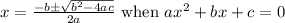
$x=\frac{-b \pm \sqrt{b^(2)-4 a c}}{2 a} \text{ when } a x^(2)+b x+c=0$

where a=1; b=-9; c=3 and x is the value to be found.

On substituting the values in the equation we get,

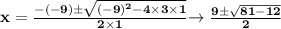
$\bold{x=(-(-9)\pm√((-9)^2-4*3*1))/(2*1)} \bold{\rightarrow (9\pm√(81-12))/(2)}}$

\bold{\Rightarrow \frac{9\pm\sqrt{69}}{2}}}

The square root of 69 is 8.3

On substituting the value in the above equation we get,

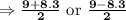
$\bold{\Rightarrow (9+8.3)/(2)}} \bold{ \text{ or }} \bold{ (9-8.3)/(2)}}$

On solving we get,

$\bold{\Rightarrow (17.3)/(2)}} \bold{ \text{ or }} \bold{ (0.7)/(2)}}$

$\bold {\Rightarrow \therefore \text{ x will be }} \bold{8.65} \bold{\text{ or }} \bold{0.35}$

Please log in or register to add a comment.