Answer:

Explanation:
A quadratic equation is given by:

The leading co-efficient is
 .
.
Given
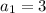 . Therefore the quadratic equation becomes:
. Therefore the quadratic equation becomes:
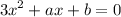 . We are to find
. We are to find
 .
.
Given the solutions of this equation are:
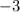 and
and
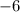 .
.
Substituting this in the equation we get:
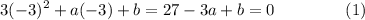
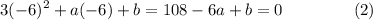
Solving
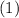 and
and
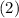 we get
we get
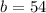 . Substituting in
. Substituting in
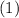 we get
we get
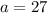 . Thus the equation becomes:
. Thus the equation becomes:
