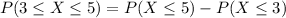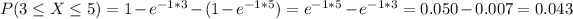Answer:
a) 1
b) 1
c)

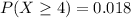
d)
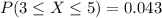
Step-by-step explanation:
An exponential distribution is a probability distribution of the time between events which occur continuously and independently at a constant average rate
 . The probability function is
. The probability function is

a) The expected value of an exponentially distributed variable X with rate
 (in this case 1) is given by
(in this case 1) is given by
![E[X]=1/\lambda](https://img.qammunity.org/2020/formulas/engineering/college/ojhui5bck72yl2mm3at7xjikmjkk8x8qud.png)
E[X] = 1/1 = 1
b) The variance of an exponentially distributed variable X with rate
 is
is
![Var[X]=1/\lambda^2](https://img.qammunity.org/2020/formulas/engineering/college/34ghti34ds2zdmyyzy07pfkdavqy65oa8n.png)
so the standard deviation is
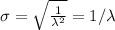
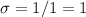
c) the cumulative distribution function is
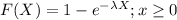
with that function we can calculate the probability of time between events be lower or equal than determined number.
So

If you need to calcuate the probability of time between events be higher than determined number you can do the following operation
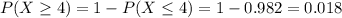
d)
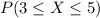 we need to make a substraction
we need to make a substraction