Question

Graph -x^2+x+6=0 and state the consecutive integers between which roots are located

Answer 1

The roots of given quadratic equation lies from
`\begin{bmatrix}-2 , 3 \end{bmatrix}`

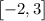
Explanation:

Given as :

The quadratic equation is x² - x -6 = 0

The quadratic equation is in form of ax² + bx +c = 0

Let x1 and x2 be the roots of equation

Sum of roots

So,
`x1 + x2 = (-b)/(a)`

And products of roots is x1 × x2 =
`(c)/(a)`

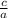
So,
`x1 + x2 = (1)/(1)`

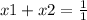
Or, x1 + x2 = 1 ......A

And x1 × x2 =
`(-6)/(1)`

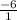
Or, x1 × x2 = - 6

Now, (x1 - x2)² = (x1 + x2)²+ 4×x1×x2

Or, (x1 - x2)² = (1)²+ 4×6

Or, (x1 - x2)² = 25

So , x1 - x2 =
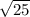`√(25)` = 5 ......B

Now solve eq A and eq B

Or, (x1 + x2) + (x1 - x2) = 1 +5

Or, 2 x1 = 6

∴ x1 = 3 And x2 = - 2

Hence The roots of given quadratic equation lies from
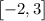`\begin{bmatrix}-2 , 3 \end{bmatrix}` Answer