Question

A man's speed with the current is 15 km/hr and the speed of the current is 2.5 km/hr. The man's speed against the current is:

Answer 1

Answer: 12.5 km/hr

Explanation:

Answer 2

10 km/h

Explanation:

It is given that a man's speed with the current is 15 km/hr and the speed of the current is 2.5 km/hr.

Man's speed with the current (Downstream) = 15 km/hr

Speed of the current = 2.5 km/hr

We need to find the man's speed against the current(Upstream).

Let x be the Man's speed against the current.

Speed of the current
`=(Downstream-Upstream)/(2)`

`2.5=(15-x)/(2)`

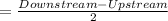
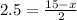
Multiply both sides by 2.

`5=15-x`

`x=15-5`

`x=10`

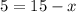
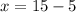
Therefore, the man's speed against the current is 10 km/hr.