Question

A gift shop sells 2000 boxes of scented candles a year. The ordering cost is $100 for scented candles, and holding cost is $10 per box per year. What is the minimum annual amount of these combined costs the gift shop could pay?

$100

$1000

$2000

$200

$1200

Answer 1

Minimun cost: $2000

Step-by-step explanation:

We solve for the optimal order size using the

Economic Order Quantity:

`Q_(opt) = \sqrt{(2DS)/(H)}`

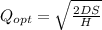
Where:

D = annual demand = 2,000 boxes

S= setup cost = ordering cost = $ 100

H= Holding Cost = $10.00

`Q_(opt) = \sqrt{(2(2,000)(100))/(10)}`

`Q_(opt) = √(40,000)`

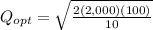
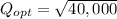
EOQ 200

It should order: 2,000 demand / 200 order size = 10 times

At a cost of 1,000 dollar (100 units x $ 10)

It will face an average inventory of 100 units thus holding cost:

100 units x 10 dollar per unit = 1,000

Total cost: 1,000 + 1,000 = 2,000