Answer:
The cost of 1 Rose Bush= $ 8
The cost of 1 Shrub = $12.
Explanation:
The cost of 11 rose bushes and 4 shrubs = $136
The cost of 2 rose bushes and 11 shrubs = $148
Let the cost of one rose bush = $x
and the cost of one shrub = $ y
Now, according to the question:
11 x + 4 y = 136
and 2 x + 11 y = 148
From (1), we get that 11x = 136 - 4y
or,
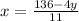
Substitute this value of x in equation (2), we get
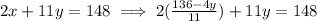
or,

or, 113 y = 1356
or, y = 1356/113 = 12
⇒ y = 12, So
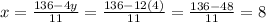
or, x =8 and y = 12 is the solution of the above system.
Hence, the cost of 1 rose bush = $x = $8
and The cost of 1 shrub = $ y = $12.