Answer:
Part 1)
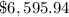
Part 2)
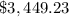
Part 3)
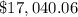
Part 4)

Part 5) The Option A is the best way to invest the money by $4,223.94 than Option B
Explanation:
Part 1)
we know that
The compound interest formula is equal to

where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
n is the number of times interest is compounded per year
in this problem we have
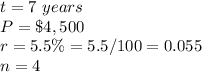
substitute in the formula above

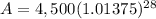
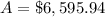
Part 2)
we know that
The formula to calculate continuously compounded interest is equal to
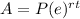
where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
e is the mathematical constant number
we have
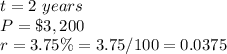
substitute in the formula above
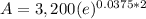
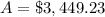
Part 3)
we know that
The compound interest formula is equal to

where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
n is the number of times interest is compounded per year
in this problem we have
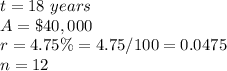
substitute in the formula above
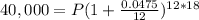

![P=40,000/[((12.0475)/(12))^(216)]](https://img.qammunity.org/2020/formulas/mathematics/high-school/i5xnz888cole62qc4kg1trkvp1hsazoaaf.png)
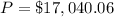
Part 4)
we know that
The formula to calculate continuously compounded interest is equal to
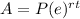
where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
e is the mathematical constant number
we have
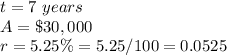
substitute in the formula above
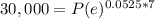
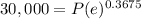
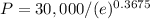

Part 5)
Option A
we know that
The compound interest formula is equal to

where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
n is the number of times interest is compounded per year
in this problem we have
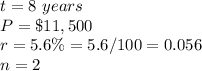
substitute in the formula above
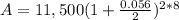
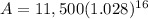
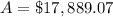
Option B
we know that
The formula to calculate continuously compounded interest is equal to
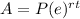
where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
e is the mathematical constant number
we have
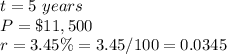
substitute in the formula above
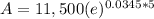
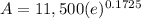

Compare the options
Option A ------>
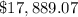
Option B ----->
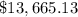
so
Option A > Option B
Find out the difference

therefore
The Option A is the best way to invest the money by $4,223.94 than Option B