Question

Find the perimeter of △ABC with vertices A(−5, −5), B(3, −5), and C(−5, 1).

Answer 1

Perimeter: 24 u

Explanation:

In order to find the perimeter of the triangle you have to find the distance between the vertices using the distance formula and add all the distances:

Distance AB

`d=\sqrt{ ( x_(2) - x_(1) )^(2) + ( y_(2) - y_(1) )^(2) } \\d=\sqrt{ ( 3 - (-5) )^(2) + ( (-5) - (-5) )^(2) } \\d=\sqrt{ ( 3+5 )^(2) + ( -5+5 )^(2) } \\d=\sqrt{ (8)^(2) + ( 0 )^(2) } \\d=√( 64 ) \\d=8`

Distance BC

`d=\sqrt{ ( x_(2) - x_(1) )^(2) + ( y_(2) - y_(1) )^(2) } \\d=\sqrt{ ( -5 - 3 )^(2) + ( 1 - (-5) )^(2) } \\d=\sqrt{ ( -8 )^(2) + ( 1+5 )^(2) } \\d=\sqrt{ (-8)^(2) + ( 6 )^(2) } \\d=√(64+36) \\d=√(100)\\d=10`

Distance CA

`d=\sqrt{ ( x_(2) - x_(1) )^(2) + ( y_(2) - y_(1) )^(2) } \\d=\sqrt{ ( -5 - (-5) )^(2) + ( 1 - (-5) )^(2) } \\d=\sqrt{ ( -5+5 )^(2) + ( 1+5 )^(2) } \\d=\sqrt{ (0)^(2) + ( 6 )^(2) } \\d=√(36) \\d=6`

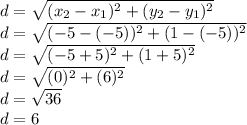
Adding all the 3 distances we have that:

P=8+10+6=24 u