Question

Find the differance between the pair of points.
(6,4) and (6,-8)

Answer 1

Difference that is distance between two given points (6, 4) and (6, -8) is 14 units.

Solution:

Need to find the difference that is distance between the two points.

Two given points are
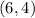`(6, 4)` and
`(6, -8)`

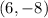
We will be using distance formula to find the distance between two points

According to the distance formula distance d between two points
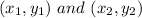`(x_1, y_1) \ and \ (x_2, y_2)` is given by

`\bold{\mathrm{d}=\sqrt{\left(x_(2)-x_(1)\right)^(2)+\left(y_(2)-y_(1)\right)^(2)}}`

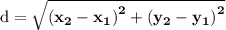
In our case
`x_1 = 6; \ y_1 = 4; \ x_2 = 6; \ y_2 = -8`

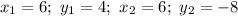
On substituting given values in distance formula we get ,

`\mathrm{d}=\sqrt{(6-6)^(2)+(-8-6)^(2)}`

`\Rightarrow \mathrm{d}=\sqrt{(0)^(2)+(-14)^(2)}`

`\Rightarrow d=√(196)`

`\Rightarrow \mathrm{d}=14`

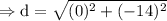
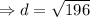
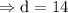
Hence, distance between two given points is 14 units.