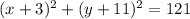Question

The center of a circle lies on the line y = 4x + 1 and is tangent to the x-axis at (−3,0) . What is the equation of the circle in standard form? Enter your answer by filling in the boxes. (x__)^2+(y__)^2=___

Answer 1

The Answer is (x + 3)^2 + (y + 11)^2 = 121

Explanation:

I took the Test

Answer 2

`(x+3)^2+(y+11)^2=121`

Explanation:

Consider a sketch of the problem as shown in the picture, where:

• Blue line is given by y = 4x + 1.
• Point B is the center of the circle.
• Point A is (-3, 0).

Since the center of the circle lies on the line y = 4x +1 and is tangent to the x-axis at point A, then its radius BA is perpendicular to the x-axis. To find the coordinates of point B, we must replace x = -3 into the blue line equation: y = 4x(-3) + 1 = -11.

So, we know that the center of the circle is at B=(-3, -11). And furthermore, the radius BA is of length r=11.

Since the general equation of the circle of radius lenght r centered at (h, k) is given by

`(x-h)^2+(y-k)^2=r^2`

then with h = -3, k = -11 and r= 11, the equation of our circle is

`(x+3)^2+(y+11)^2=121`