Answer: The maximum possible weight of the lightest box would be 3.
Explanation:
Since we have given that
Average of three boxes = 7
Let the weights of three boxes be 'x', 'y', 'z'.
So, we get
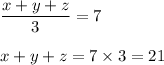
Median = 9
Since it has odd number of boxes i.e. 3
So, median would be the middle term i.e. b = 9
So, it becomes
a,9,c
Suppose c at its highest i.e. c= 9
Then it becomes,
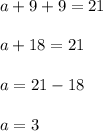
Hence, the maximum possible weight of the lightest box would be 3.