Question

A supervisor records the repair cost for 23 randomly selected stereos. A sample mean of $91.82 and standard deviation of $16.84 are subsequently computed. Determine the 98% confidence interval for the mean repair cost for the stereos. Assume the population is approximately normal.

Find the critical value that should be used in constructing the confidence interval. Round your answer to three decimal places.

Answer 1

Answer: 2.508

Explanation:

As per give , we have

Sample size : n= 23

Degree of freedom : df= n-1=22

significance level :
`\alpha: 1-0.98=0.02`

Since , the sample size is small (n<30) and population standard deviation is unknown.

So , we use t-test.

For confidence interval , we find two-tailed test value.

Critical t-value :
`t_(\alpha/2, df)=t_(0.01,22)`

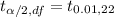
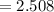`=2.508` [using students's t-critical value table]

Hence, the critical value that should be used in constructing the confidence interval = 2.508