Answer:
The points (-2, 0) and (3,5) are the ONLY solutions of the given sets of equations.
Explanation:
Here, the given equations are:
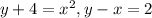
Now checking for the given points:
(a) (-2, 0)
Here,

Hence, (-2, 0) is the solution of the given equations.
b) Checking for (2,0), as (-2, 0) is a solution as shown above
Here,
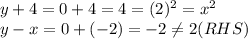
Hence, (2, 0) is NOT the solution of the given equations.
c) Checking for (3,5), as (-2, 0) is a solution as shown above
Here,

Hence, (3,5), is the solution of the given equations.
Hence, the points (-2, 0) and (3,5) are the ONLY solutions of the given sets of equations.