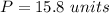Question

ASAP Please.What is the perimeter of triangle ABC? Round each step to the nearest tenth. Enter your answer in the box.

Answer 1

The perimeter of triangle ABC is 15.8 units

Explanation:

we know that

The perimeter of a triangle is equal to the sum of its three length sides

`P=AB+BC+AC`

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

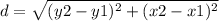
we have the coordinates

`A(5,-1),B(-1,1),C(0,-3)`

step 1

Find the distance AB

we have

`A(5,-1),B(-1,1)`

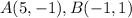
substitute in the formula

`d=\sqrt{(1+1)^(2)+(-1-5)^(2)}`

`d=\sqrt{(2)^(2)+(-6)^(2)}`

`d_A_B=√(40)\ units`

`d_A_B=6.3\ units`

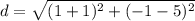
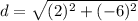
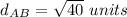
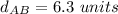
step 2

Find the distance BC

we have

`B(-1,1),C(0,-3)`

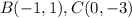
substitute in the formula

`d=\sqrt{(-3-1)^(2)+(0+1)^(2)}`

`d=\sqrt{(-4)^(2)+(1)^(2)}`

`d_B_C=√(17)\ units`

`d_B_C=4.1\ units`

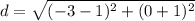
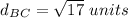
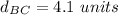
step 3

Find the distance AC

we have

`A(5,-1),C(0,-3)`

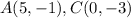
substitute in the formula

`d=\sqrt{(-3+1)^(2)+(0-5)^(2)}`

`d=\sqrt{(-2)^(2)+(-5)^(2)}`

`d_A_C=√(29)\ units`

`d_A_C=5.4\ units`

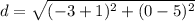
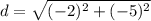
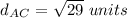
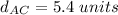
step 4

Find the perimeter

`P=AB+BC+AC`

substitute the values

`P=6.3+4.1+5.4`

`P=15.8\ units`