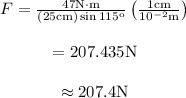Question

he tune-up specifications of a car call for the spark plugs to be tightened to a torque of 47 N⋅m . You plan to tighten the plugs by pulling on the end of a 25-cm-long wrench. Because of the cramped space under the hood, you'll need to pull at an angle of 115 ∘ with respect to the wrench shaft. You may want to review (Page

Answer 1

207.4 N

Step-by-step explanation:

The torque
`\tau` on a body is

`\tau = r* F` where r is the radius vector from the point of rotation to the point at which force F is applied.

The product of r and F is equal to the product of magnitude of r and F multiplied by the sine of angle between both vectors.

Therefore, torque is also given by

`\tau = rF\sin \theta`

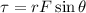
Where
`\theta` is the angle between r and F.

Use the expression of torque.

Substitute L for r in the equation
`\tau = rF\sin \theta`

`\tau = LF\sin \theta`

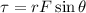
Where L is the length of the wrench.

Making F the subject

`F = \frac{\tau }{{L\sin \theta }}`

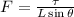
Force required to pull the wrench is given as,

`F = \frac{\tau }{{L\sin \theta }}`

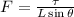
Substitute
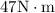`47{\rm{ N}} \cdot {\rm{m}}` for
`\tau`, 25 cm for L, and 115o for
`\theta`

`\begin{array}{c}\\F = \frac{{47{\rm{ N}} \cdot {\rm{m}}}}{{\left( {25{\rm{ cm}}} \right)\sin {{115}^{\rm{o}}}}}\left( {\frac{{1{\rm{ cm}}}}{{{{10}^( - 2)}{\rm{ m}}}}} \right)\\\\ = 207.435{\rm{ N}}\\\\ \approx 207.4{\rm{ N}}\\\end{array}`