Answer:
FALSE
Explanation:
The central limit theorem tell us that for a random sample of size n, the average of the sample will be approximately normally distributed with mean
 and variance
and variance
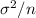 , where
, where
 and
and
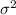 are the mean a variance respectively of the sampling distribution. This always that the mean and the variance are both finite and the sample size n is greater than 30. We could use the central limit theorem, but in this case does not help us because we are considering 25 randomly selected data values, besides, we do not know the distribution of the original population.
are the mean a variance respectively of the sampling distribution. This always that the mean and the variance are both finite and the sample size n is greater than 30. We could use the central limit theorem, but in this case does not help us because we are considering 25 randomly selected data values, besides, we do not know the distribution of the original population.