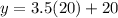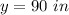Question

you were 20 inches tall at birth, and 48 inches tall on your 8th birthday. Create a linear equation to represent this equation. According to your equation, how tall will you be at age 20?

Answer 1

Part a)
`y=3.5x+20`

Part b)
`90\ in`

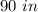
Explanation:

Part a) Create a linear equation to represent this situation

Let

x -----> the number of years

y ----> the height in inches

we have the points

(020) ----> at birth the height was 20 inches

(8,48) ---> 8th birthday the height was 48 inches

step 1

Find the linear equation in slope intercept form

`y=mx+b`

where

m is the slope

b is the y-coordinate of the y-intercept

Find the slope m

`m=(48-20)/(8-0)\\m=3.5\ in/year`

Find the value of b

`b=20\ in` ----> the point (0,20) is the y-intercept

substitute

`y=3.5x+20`

Part b) According to your equation, how tall will you be at age 20?

For x=20 years

substitute the value of x in the linear equation and solve for y

`y=3.5(20)+20`

`y=90\ in`