Answer:
resistance R = 1.717 ×
 Ω
Ω
diameter is 5.484 ×
 m
m
Step-by-step explanation:
given data
length = 2.4 m
cross section = 6.2 mm
solution
we know resistance formula that is
resistance R =
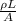 ............1
............1
here ρ is resistivity and A is cross section and L is length
so put her value
aluminum resistance R =
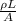
resistance R =

resistance R = 0.001717 = 1.717 ×
 Ω
Ω
and
copper resistance R =
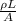
resistance R =
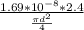
1.717 ×
 =
=
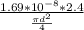
solve we get
d = 0.005484 = 5.484 ×
 m
m
so diameter is 5.484 ×
 m
m