Question

Assume that the risk-free rate is 8 percent, the expected return on the market is 13 percent, and that a share of stock in your company has a beta of 1.4. If the current dividend just paid (D0) was $2.25 and is expected to grow at a long-run growth rate of 10 percent per year, then how much should investors be willing to pay for this stock? Group of answer choices

Answer 1

The investors should be willing to pay $49.50 for this stock

Step-by-step explanation:

Hi, first, we need to find out what the cost of equity is in order to find the price of the stock. that is:

`r(e)=rf+beta(rm-rf)`

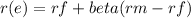
Where:

rf= Risk free rate

rm=return on the market

r(e)=cost of equity

After finding r(e), we would need to find the price using the following equation.

`Price=(Do(1+g))/(r(e)-g)`

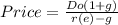
Where:

Do= last dividend

g= growth rate

r(e)= cost of equity.

ok, so, let´s find out what the cost of equity is.

`r(e)=0.08+1.4(0.13-0.08)=0.15`

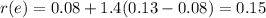
So, the r(e)=15%, now let´s find the price of this stock

`Price=(2.25(1+0.1))/(0.15-0.10) =49.50`

Therefore, the price of this stock is $49.50

Best of luck.