Question

If sin 0 equals -4/5 and 0 is in quadrant 4 the value of sec 0 is​

Answer 1

bearing in mind that the hypotenuse is never negative, since it's just a distance unit from the center of the circle, we know the sine is -(4/5), since the hypotenuse is positive, then the negative must be the 4, or -4/5.

we know the angle θ is in the IV Quadrant, where the x/adjacent is positive and the y/opposite is negative, so

`\bf sin(\theta )=\cfrac{\stackrel{opposite}{-4}}{\stackrel{hypotenuse}{5}}\qquad \impliedby \textit{let's find the \underline{adjacent side}} \\\\\\ \textit{using the pythagorean theorem} \\\\ c^2=a^2+b^2\implies \pm√(c^2-b^2)=a \qquad \begin{cases} c=hypotenuse\\ a=adjacent\\ b=opposite\\ \end{cases}`

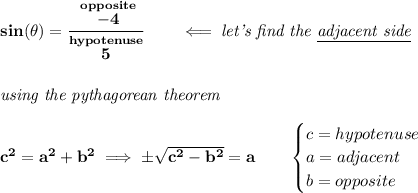
`\bf \pm√(5^2-(-4)^2)=a\implies \pm√(25-16)=a\implies \pm 3=a\implies \stackrel{IV~Quadrant}{+3=a} \\\\[-0.35em] ~\dotfill\\\\ ~\hfill sec(\theta )= \cfrac{\stackrel{hypotenuse}{5}}{\stackrel{adjacent}{3}}~\hfill`