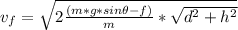Question

A block of mass mm starts from rest and slides down from the top of a wedge of height hh and length dd. The surface of the wedge forms an angle of \thetaθ with respect to the horizontal direction. The force of kinetic friction between the block and the wedge is \vec{f} f ​ . How fast is the block traveling when it reaches the bottom of the wedge?

Answer 1

`v_f = \sqrt{2((m*g*sin\theta - f))/(m)*√(d^2 + h^2) }`

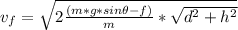
Step-by-step explanation:

Known data

m= mass of the block

h= high of the wedge

d= length of the wedge

θ = angle θ of the wedge with respect to the horizontal direction

f = force of kinetic friction between the block and the wedge

g = 9.81 m/s² : acceleration due to gravity

Newton's second law:

∑F = m*a Formula (1)

∑F : algebraic sum of the forces in Newton (N)

m : mass in kilograms (kg)

a : acceleration in meters over second square (m/s²)

We define the x-axis in the direction parallel to the movement of the block on the wedge and the y-axis in the direction perpendicular to it.

Calculated of the weight

W= m*g

x-y weight components

Wx= Wsin θ= m*g*sin θ

Wy= Wcos θ =m*g*cos θ

We apply the formula (1) to calculated acceleration of the block:

∑Fx = m*ax , ax= a : acceleration of the block

Wx-f = m*a

`a= (W_(x)-f )/(m) = (m*g*sen\theta-f )/(m)`

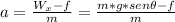
Kinematics of the block

Because the block moves with uniformly accelerated movement we apply the following formula to calculate the final speed of the block :

vf²=v₀²+2*a*X Formula (2)

Where:

X:displacement

v₀: initial speed

vf: final speed

a: acceleration

Data

v₀=0

`a= (m*g*sen\theta-f )/(m)`

`X=\sqrt{d^(2)+h^(2)  }`

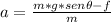
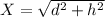
We replace data in the formula (2)

vf²=v₀²+2*a*X

vf²=0+2*a*X

`v_f = \sqrt{2((m*g*sin\theta - f))/(m)*√(d^2 + h^2) }`