Answer:
3.731 minutes
Step-by-step explanation:
Let the amount of salt in the tank at any time be x(t)
Since x(0)=5 g is dissolved in 20 liters of water
Brine with 2 grams per liter salt enters the tank at the rate of 3 liters/min
Salt entering per minute is 2* 3=6 grams/min
Volume of liquid leaving the tank is the same as the volume of liquid of tank entering, 3 liters/min
volume of liquid remains at 20 liters at all times
At any given points of time, the concentration of salt is
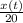 grams/liter
grams/liter
Amount of liquid leaving per minute is 3 liters/min so that the amount of salt leaving is
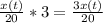 grams/minute
grams/minute
Differential equation governing the salt amount in the tank is
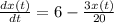
Therefore,
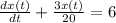
Integrating factor is
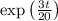 and so the equation becomes
and so the equation becomes
![(d)/(dt)\left[\exp\left((3t)/(20) \right )x(t) \right ]=6\exp\left((3t)/(20) \right )](https://img.qammunity.org/2020/formulas/physics/college/kjj4hj8c7zuo57gw4yllkue52ipdr0fqx2.png)
Therefore,
![\left[\exp\left((3t)/(20) \right )x(t) \right ]=\int 6\exp\left((3t)/(20) \right )=40\exp\left((3t)/(20) \right )+C](https://img.qammunity.org/2020/formulas/physics/college/2zhqb3ekcpgwp49q5c4i87wlyltsuryaiv.png)
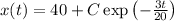
Using the initial condition
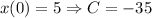
 is the amount of salt at any point of time
is the amount of salt at any point of time
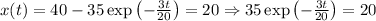
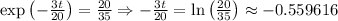

After approximately 3.731 minutes, we have 20 grams of salt in the tank