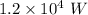Question

A 700 kg elevator starts from rest. It moves upward for 4.50 s with constant acceleration until it reaches its cruising speed, 1.75 m/s. (a) What is the average power of the elevator motor during this period? W (b) How does this power compare with the motor power when the elevator moves at its cruising speed? Pcruising = W

Answer 1

(a). The average power of the elevator motor during this period is 6235.25 W.

(b). The power input from motor is
`1.2*10^(4)\ W`

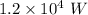
Step-by-step explanation:

Given that,

Mass of elevator =700 kg

Initial velocity = 1.75 m/s

Time = 4.50 s

We need to calculate the acceleration of the elevator

Using formula of acceleration

`a = (v)/(a)`

Put the value into the formula

`a=(1.75)/(4.50)`

`a=0.38\ m/s^2`

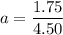
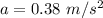
We need to calculate net force on elevator

Using formula of net force

`T=mg+ma`

`T=m(g-a)`

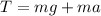
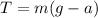
Put the value into the formula

`T=700(9.8+0.38)`

`T=7126\ N`

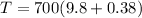
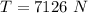
We need to calculate the average velocity

Using formula of average velocity

`v'=(v_(f)-v_(i))/(2)`

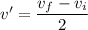
Put the value into the formula

`v'=(1.75-0)/(2)`

`v'=0.875\ m/s`

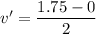
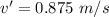
(a). We need to calculate the average power of the elevator motor during this period

Using formula of power

`P=T* v'`

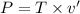
Put the value into the formula

`P=7126*0.875`

`P=6235.25\ W`

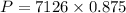
(b). We need to calculate power input from motor

Using formula of power

`P=F* v`

`P=mg* v`

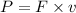
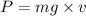
Put the value into the formula

`P=700*9.8*1.75`

`P=1.2*10^(4)\ W`

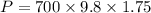
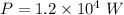
Hence, (a). The average power of the elevator motor during this period is 6235.25 W.

(b). The power input from motor is
`1.2*10^(4)\ W`

Answer 2

Given

Mass of the elevator , m = 700 kg

Initial velocity of elevator is , vi = 0 m/s

Time taken , t = 4.50 s

Speed of the elevator is, v = 1.75 m/s

Acceleration of elevator is ,
`a = (v)/(t)`

`a = (1.75)/(4.5)`

a = 0.389 m/s²

a) Net force on elevator is

T = mg + ma = m (g +a)

T = 700 kg( 9.8+0.389)

T = 7132.3 N

Average velocity is , v' = 1.75 /2 = 0.875 m/s

Average power , P = T v' = 7132.3 × 0.875 m/s

P = 6240.76 W

b) Power input from motor is

P = F x v = mg x v

= 700 x 9.8 * 1.75 m/s

P = 12005 W