Answer:
(a). The kinetic energy is 5.33 J
(b). The maximum kinetic energy is 5.33 J.
Step-by-step explanation:
Given that,
Mass of block = 1.5 kg
Suppose the force is

(a). We need to calculate the kinetic energy of the block
Using work energy theorem
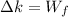

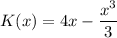 ....(I)
....(I)
Now put the value of x in equation
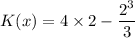
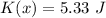
The kinetic energy is 5.33 J
For K to be minimum
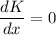

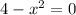

(b). We need to calculate the maximum kinetic energy of the block
For K to be maximum
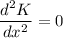
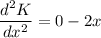
Put the value of x
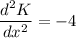
Now put the value of x in equation (I)
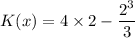
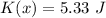
The maximum kinetic energy is 5.33 J
Hence, (a). The kinetic energy is 5.33 J
(b). The maximum kinetic energy is 5.33 J.