Answer:
14 climbers.
Explanation:
Let us assume that the number of climbers in the group is x and the cost per person on the bus is y.
Now, total cost of bus fair = 280 = xy - 0.25x + 12
{Where $12 is the cost of group permit and $0.25 per person is deducted as discount}
⇒xy - 0.25x = 268
⇒
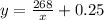
Now, it is given that y ≤ 20
⇒

⇒
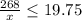
⇒
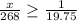
⇒
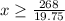
⇒
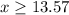
Since x can not be a fraction.
Therefore, there must be at least 14 climbers in the group for the cost per person to be $20 or less. (Answer)