Answer:
We should obtain 19 newspapers to maximize the expected profits.
Step-by-step explanation:
In this problem we have to optimize the quantity of newspaper we buy to maximize the profit.
We can divide the analysis in two parts:
1) If the amount of newspaper sold (S) is more than the newspaper we bougth (B), the profit is
 .
.
This occurs with probability
 , as S is uniformly distributed.
, as S is uniformly distributed.
2) If the amount of newspaper sold (S) is less than the newspaper we bougth (B) we have a profit of:
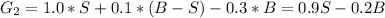
This happens with a probability of
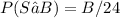 .
.
As we have S in our profit equation, we have to calculate the expected value of S to estimate the profit in function of B.
If S is smaller than B, then it is uniformly distributed between 0 and B. The expected value can be calculated as:
![E[S]=(B-0)/(2) =B/2](https://img.qammunity.org/2020/formulas/business/college/86190ff8zq03ddr5urm5qka4r4ygea54oo.png)
Then, the expected profit formula for S<B is

The total profit tcan be calculated by adding the two parts analized previously: when S>B and when S<B, affected by the probability of occurrence:
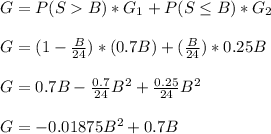
To maximize the profit G, we derive and equal zero

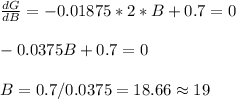
We should obtain 19 newspapers to maximize the expected profits.